隨著國際互聯(lián)網(wǎng)的飛速發(fā)展，網(wǎng)絡(luò)安全已成為現(xiàn)代信息安全研究的關(guān)鍵問題,迫切需要新的安全的信息傳輸技術(shù),。混沌是一種普遍存在于從宏觀到微觀非線性系統(tǒng)內(nèi)在的無規(guī)則而不穩(wěn)定的運(yùn)動狀態(tài)[1],。混沌系統(tǒng)也是一種復(fù)雜的非線性,、非平衡的動力過程,，其特點(diǎn)有：(1)混沌動力學(xué)特性對初始條件具有敏感依賴性。初始條件略有差別或者微小的擾動都會導(dǎo)致系統(tǒng)的最終狀態(tài)出現(xiàn)巨大的差別,，其長期演化行為不可預(yù)測,；(2)系統(tǒng)由完全確定性的方程描述，無需附加任何因素,，系統(tǒng)仍表現(xiàn)出類隨機(jī)行為,。混沌的這些特性使得基于混沌控制系統(tǒng)的加密方法具有很高的安全性[2],。
    人工神經(jīng)網(wǎng)絡(luò)是一種基于連接概念的智能模擬方法,。它具有分布式存儲信息、容錯性和大規(guī)模并行處理結(jié)構(gòu)的特點(diǎn),，并具有自適應(yīng),、自學(xué)習(xí)、自組織的能力,。在理論上能夠?qū)W習(xí)并以任意精度逼近任何非線性和不確定系統(tǒng)的動力學(xué)模型,。人工神經(jīng)網(wǎng)絡(luò)為解決混沌非動力系統(tǒng)加密方法提供了新的方法和思路[3]。
    小波神經(jīng)網(wǎng)絡(luò)是近年來新興的一種數(shù)學(xué)建模分析方法,，是結(jié)合小波變換和傳統(tǒng)人工神經(jīng)網(wǎng)絡(luò)的思想而形成的,。小波神經(jīng)網(wǎng)絡(luò)充分繼承了小波分析與神經(jīng)網(wǎng)絡(luò)兩者的優(yōu)點(diǎn)，具有更靈活有效的函數(shù)逼近能力和較強(qiáng)的容錯能力,，可以有效地克服普通人工神經(jīng)網(wǎng)絡(luò)模型所固有的一些缺陷,。本文介紹了小波神經(jīng)網(wǎng)絡(luò)的模型和算法,提出一種基于小波神經(jīng)網(wǎng)絡(luò)的混沌加密方法。通過實(shí)驗(yàn)仿真,，證實(shí)了該方法的可行性,。
1 小波神經(jīng)網(wǎng)絡(luò)模型
    小波神經(jīng)網(wǎng)絡(luò)是在小波分析的基礎(chǔ)上提出的一種前饋神經(jīng)網(wǎng)絡(luò)，它可以被認(rèn)為是RBF網(wǎng)絡(luò)的推廣。本文

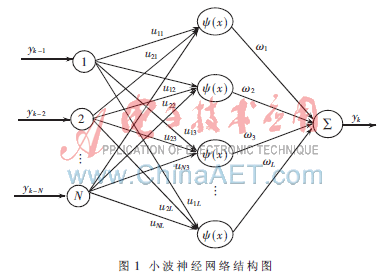
    對于輸入的樣本對,，目的是確定網(wǎng)絡(luò)參數(shù)uki,、?棕i、ak,、bk和L,，使得f(k)與y(k)兩序列的擬合最優(yōu)。網(wǎng)絡(luò)參數(shù)可以通過最小均方誤差能量函數(shù)進(jìn)行優(yōu)化：

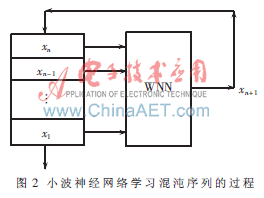
3 基于小波神經(jīng)網(wǎng)絡(luò)的混沌加密算法
3.1 基于小波神經(jīng)網(wǎng)絡(luò)的混沌加密過程
    (1)選取已知的混沌序列樣本,，將這些樣本作為小波神經(jīng)網(wǎng)絡(luò)的學(xué)習(xí)樣本,，確定小波神經(jīng)網(wǎng)絡(luò)的權(quán)值uki、?棕i,并將其通過安全信道傳送給信息接收方,。
　

　 使用學(xué)習(xí)后的小波神經(jīng)網(wǎng)絡(luò)給馬?。返陆稹段矣幸粋€夢想》中的第2段進(jìn)行加密操作。當(dāng)混沌初值為0.1時,，小波神經(jīng)網(wǎng)絡(luò)加密后的序列為：
    egbvsgaarh Iy cseywatrosj  ri cemieoe aNootabmpoec s.ei i cn ri nhm iegarfrtego  ,icodm t hni u wt at i lo anoarPcsysn sf b mi tefdt .h hssvoooatce o epr dastaioalee ,wtaer go voh todb s tdh huyga tneitoskweh.osatao j n eidehon eih nle aoryerdl atfe, e Aod si  cc nass Ta ngiagciwtlfFmn nnera ml t  pdh m Eemesenaigac ahusa  ete lieiyn
    當(dāng)混沌初值為0.100 5時,，小波神經(jīng)網(wǎng)絡(luò)加密后的序列為：
    asce ne olsn o.d ver u yhr mrth  gelTeeiubaeiaiawy t om ga  s s ssy ardnatjae t  ie j ea l   anoshi,naaer osytA.i i ocoeygo e atirhcartrgcselehdh h seodnelniyenvaioosm c etoa h gtiioaeia,tot  ofnamhncbc nwiPteime.Eg  emave mamufgs tothsnaIpoc eworsstn ns t gcowha ,f ke cdp  bto n gdispd oel F hmotNm lbntaw e o adoeirad erfi fcsho eii
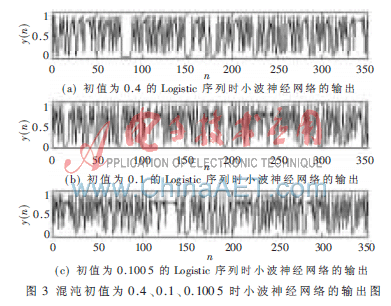
    混沌初值發(fā)生微小的改變，小波神經(jīng)網(wǎng)絡(luò)產(chǎn)生性質(zhì)完全不同的混沌密文序列,。解密后的明文序列與明文均為：
    Five score years ago, a great American, in whose symbolic shadow we stand today, signed the Emancipation Proclamation. This momentous decree came as a great beacon light of hope to millions of Negro slaves who had been seared in the flames of withering injustice. It came as a joyous daybreak to end the long night of their captivity.
    當(dāng)混沌初值分別為0.4,、0.1和0.100 5時，分別用小波神經(jīng)網(wǎng)絡(luò)得到混沌加密序列,。圖4為三種混沌初值分別用小波神經(jīng)網(wǎng)絡(luò)得到混沌序列的自相關(guān)函數(shù),。由圖4可知，基于小波神經(jīng)網(wǎng)絡(luò)產(chǎn)生的混沌序列具有良好的相關(guān)性,，可以滿足密碼學(xué)的要求,。
    本文提出一種基于小波神經(jīng)網(wǎng)絡(luò)的混沌序列生成方法，并基于該方法提出一種新的混沌加密方案,。通過計(jì)算機(jī)仿真表明,，該方法通過小波神經(jīng)網(wǎng)絡(luò)可產(chǎn)生比單一混沌映射更多的、性能更接近理論值的混沌序列,。同時基于該模型的混沌加密方案具有高度的保密性和靈敏性,。

參考文獻(xiàn)
[1] 譚文，王耀南.混沌系統(tǒng)的模糊神經(jīng)網(wǎng)絡(luò)控制理論與方法[M].北京：科學(xué)出版社,2008.
[2] 劉年生,，郭東輝,，吳伯僖.混沌神經(jīng)網(wǎng)絡(luò)及其在保密通信中的應(yīng)用[J].電訊技術(shù)，2002(5):141-147.
[3] 鄒阿金,，肖秀春.基于混沌控制系統(tǒng)的神經(jīng)網(wǎng)絡(luò)異步加密[J]. 計(jì)算機(jī)工程,，2008，34(12):160-161.
[4] 張德豐.MATLAB神經(jīng)網(wǎng)絡(luò)應(yīng)用設(shè)計(jì)[M].北京：機(jī)械工業(yè)出版社,，2009.
[5] 趙嘉莉，羅四維，溫津偉.基于神經(jīng)網(wǎng)絡(luò)的混沌加密算法[J].計(jì)算機(jī)研究與發(fā)展,，2001,，38(12):1475-1479.